
We've already taken a first look at symbolic differential equation solvers in the context of simple IVPs of the form ${\displaystyle \left\{\begin{array}{l}\frac{dy}{dx}=f(x)\\y(0)=y_0\end{array}\right.}$. This page applies the same methods to solve some less trivial separable differential equations.
Let's feed dsolve() the differential equation that models any function whose rate of change is proportional to its value.
>> syms k y0 t y(t) >> dsolve( diff(y)==k*y, y(0)==y0 ) ans = y0*exp(k*t)
MATLAB has produced the general solution.
Here we give dsolve() the equation ${\displaystyle \frac{dy}{dx}=(1+y)e^x}$ without any side conditions.
>> syms x y(x) >> dsolve( diff(y)==(1+y)*exp(x) ) ans = C4*exp(exp(x)) - 1
MATLAB has returned a one-parameter family of solutions to the differential equation. C4 represents an arbitrary constant.
The next example shows that we don't necessarily need to solve our differential equation algebraically for $\frac{dy}{dx}$ before passing it to dsolve().
>> syms x y(x) >> dsolve( y*(x+1)*diff(y)==x*(y^2+1) ) ans = (exp(C7 + 2*x - 2*log(x + 1)) - 1)^(1/2) -(exp(C7 + 2*x - 2*log(x + 1)) - 1)^(1/2) i -i
If the differential equation above modelled a necessarily real-valued quantity $y$, then of course the non-real singular solutions $\pm i$ could be dismissed. It may appear that the interesting pair of solutions is only valid for $x>-1$. Note, however, that MATLAB's log() is a complex extension of the natural log function. This complex log is not only defined for negatives, it also outputs a constant imaginary part for negative inputs which may be cancelled by setting the imaginary part of C7 appropriately. So in fact MATLAB's solution set includes real-valued solutions on $x<-1$. Of course examining the differential equation itself quickly reveals that there can't be a real-valued solution on any interval that includes $x=-1$.

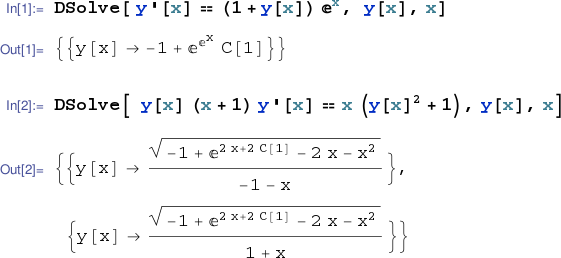
Notice that in the last example Mathematica did not produce the singular solutions $y=\pm i$.